How to do a topography assessment?

Introduction

If you design, build or own a telecom mast or a tower, the chance that you have to carry out a topography assessment is very high. This is specially valid if you have to assess an existing structure. While many towers in the field were rolled-out as a result of certain projects where likely the towers were grouped according to some common parameters. These could be wind speed, antenna EPA area, topography category or other factors. However, it not necessarily mean that one particular tower is deployed to the proper topography category. In my practise I have seen many masts which was deployed on hill, while the design parameters were set as it was on a flat terrain. This scenario brings a potentially great risk to the named structure. What we can do? Well, design standards are very clear how to make a topography assessment, or orography in Eurocode terms.

In this post I will guide you through one approach how to carry out a topography assessment as per the TIA-222-H code. By the end of this post you will have a better understanding about the following:

• How to carry out a topography assessment?
• Why it is so important?
• How to interpret the design standard requirements?
• How to use an automated workflow to eliminate the manual work?

Preparation

First things, first. We have to gather somehow the elevation profile of our selected site in subject to be able to carry out the topography assessment. This can be achieved in many ways but here I will demonstrate one solution, by using Google Earth application.

In case you are not familiar with Google Earth Pro, I've created a small screencast where you can follow the steps to create an elevation profile. See below. If you are familiar with it, you can jump right away to the chapter Topography Assessment.

Topography assessment

Choose a standard

Even we use TIA-222 w still need to decide which version to follow. The version "H" is effective since the 1st of January 2018, still many folks use and more familiar with the "G" version. This is very normal and in general takes time for practicing engineers to shift from one code to another. We, at TowerUp are aiming to be to forefront, so we early one shifted to the TIA-222-H version wherever it was applicable and allowable by customers. So in this post I'll follow the TIA-222-H version but I will highlight differences compared to the "G".

Wind speed-up over hills, ridges and escarpment

We have to consider speed-up effect according to TIA-222-H under the following conditions:

1. The hill, ridge or escarpment is isolated and unobstructed by other similar topographic features of comparable height for a radius of 2 miles [3.22 km] or 100 times of the height of the feature, whichever is less, measured horizontally from the crest, and
2. The hill, ridge or escarpment protrudes by a factor of two or more above the average height of the surrounding upwind terrain features within a 2 mile [3.22 km] radius. The slope (vertical to horizontal ratio) of the topographic feature exceeds 0.10, and
3. The height of the topographic feature is greater than or equal to 15 ft. [4.57 m] for Exposure C, D, or Site-Specific Exposures; and 60 ft. [18 m] for Exposure B, and
4. The structure is located on the upper half of the hill, ridge or escarpment.

As you can see from here, all the above mentioned conditions need to be fulfilled. If one of these conditions are not met, then we can proceed with our design with topogpraphic factor $K_{zt}=1.0$.

Choose procedure

We have three options to choose when it comes to topographic method acc. to TIA-222-H chapter 2.6.6.2 Topographic Factor Procedures.

• Method 1: Simplified Topographic Factor Procedure
• Method 2: Rigorous Topographic Factor Procedure
• Method 3: Site-specific Topographic Factor Procedure

In this post I'll guide you through both Method 1 and Method 2.The Rigorous Topographic Factor Procedure doesn't exist in TIA-222-G, as that version contains only one method, which is identical to Method 1 in the newer code.

Method 1

As a first step we have to categorize our topographic feature and find the best fit within the following 4 options:

• Category 1: No abrupt changes in general topography, e.g. flat or rolling terrain, no wind speed-up consideration shall be required. $(K_{zt}=1.0)$
• Category 2: Structures located at or near the crest of an escarpment. Wind speed-up shall be considered to occur in all directions. Structures located vertically on the lower half of an escarpment or horizontally beyond 8 times the height of the escarpment from its crest, shall be permitted to be considered as Topographic Category 1.
• Category 3: Structures located in the upper half of a hill. Wind speed-up shall be considered to occur in all directions.
• Category 4: Structures located in the upper half of a ridge. Wind speed-up shall be considered to occur in all directions.

We can see that from the standard's descriptions that basically we have to choose from flat, escarpment, hill or ridge as type of the feature. These feature types are coming from wind tunnel tests where it was demonstrated how speed-up effects occur when a wind-flow approaches them. It is beyond the scope of this post to explain in more details why and how the different topographic features influences the wind-flow. However, after several site-specific assessment one thing we can understand from the design ridges are creating the biggest speed-up effects. There are other industries where this comes very handy (better to say, a "must"), that is the aviation industry. You can think of a ridge as a wing of an aircraft. Those speed-up effects are responsible to keep us air-born while we are cruising on a passenger aircraft. More precisely, the vertical component of the speed-up effect which we will not discuss here. For structural designs of tall structures we are more concerned about the lateral forces, so the horizontal effects of the wind speed-up.

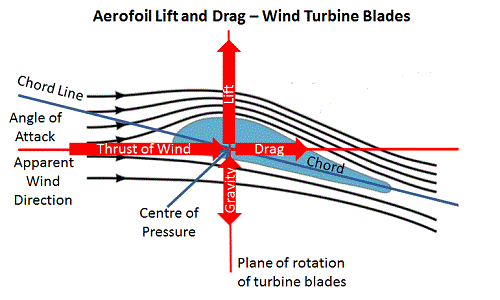
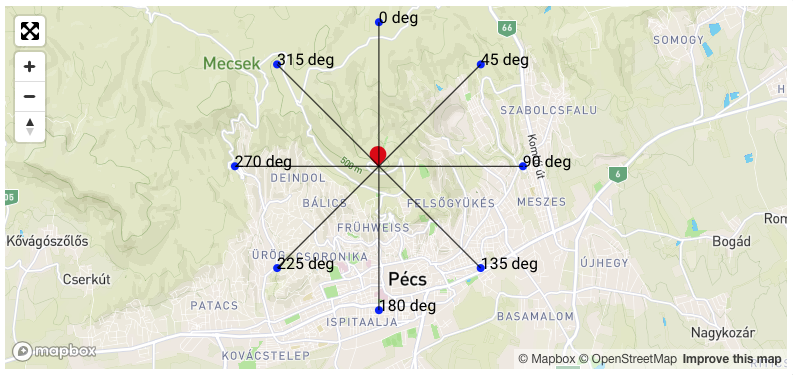
Back to our exercise, we need to have all 8 elevation profiles, in the each 45 degree sectors. One can say that why it is not enough to make only 4 profiles, as the identical paths are only 4? That is true, however, unfortunately we cannot skip to proceed with all 8 and I explain it in a second. While the 4 paths are identical, the direction of the wind where it is blowing are not. According to the design code we have to consider up-wind slope and down-wind slope. This means that one elevation profile, let say North-South (NS), likely has different topographic on the norther part than on the southern part. A perfect escarpment by having the location at the crest shows a topographic feature from the left side and being completely flat on the right hand side. That's why we cannot skip to the only 4 paths.

The goal of this guide is to demonstrate the steps how to carry out a topography assessment, so I'll not detail all 8 elevation profiles but rather focus on the task which can be repeated for all directions. In practise, you will create all 8 directions and then you can make your judgment which type of topographic feature are you assessing. If we would have done all 8 directions, then we could arrive to the conclusion that our topography feature is a hill. If you know mapping or a little bit experienced in GIS, then you can see the trajectory or iso lines for the altitudes. One can also argue that this topography feature is more a ridge, but I will show you why I think this still a hill.   At the end of this post we will discuss how to carry out this assessment using an automated procedure at the TowerUp platform which underlying algorithm also detects the type of the topography features. But for now, below is an outline how your paths would look like.

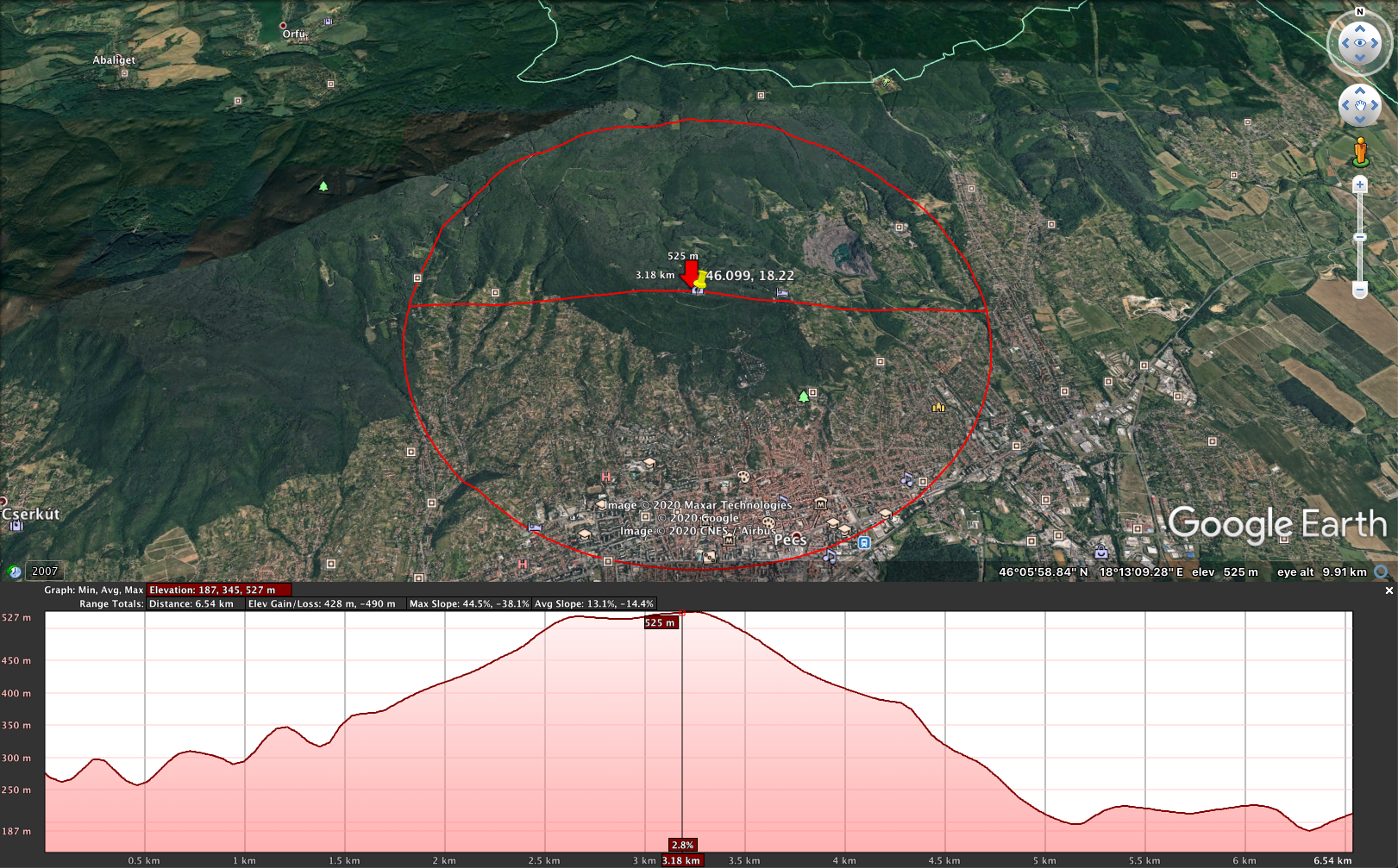
Elevation profile from Google Earth Pro

As in the screen cast I've demonstrated how to create an elevation profile, the following screen shot was made.

From here, we can proceed to collect some parameters which we need for our calculation. When we say perform a topography assessment we actually mean to calculate the topography factor, $K_{zt}$. The formula to calculate $K_{zt}$ looks like this:
$$ K_{zt}=[1+\frac{K_cK_t}{K_h} ]^2 $$
where:
$K_c = $ terrain constant given in table 2-4
$K_t = $ topographic constant given in Table 2-5
$K_h = $ height reduction factor given by the following equation:
$$ = e^{ ( \frac{f \cdot z}{H} )} $$
$e = $ natural logarithmic base = 2.718
$f = $ height attenuation factor given in Table 2-5
$z = $ height above ground level at the base of the structure
$H = $ height of crest above surrounding terrain

In order to be able to proceed, we need to understand what "H" exactly means in this equation. What is actually surrounding terrains? Well, surrounding terrain means the altitude of the average upwind terrain excluding our topography feature. I mentioned before that it is important to differentiate the upwind and downwind slopes. So we need to use the upwind segment of the terrain, but I will also show the downwind terrain just for completeness.

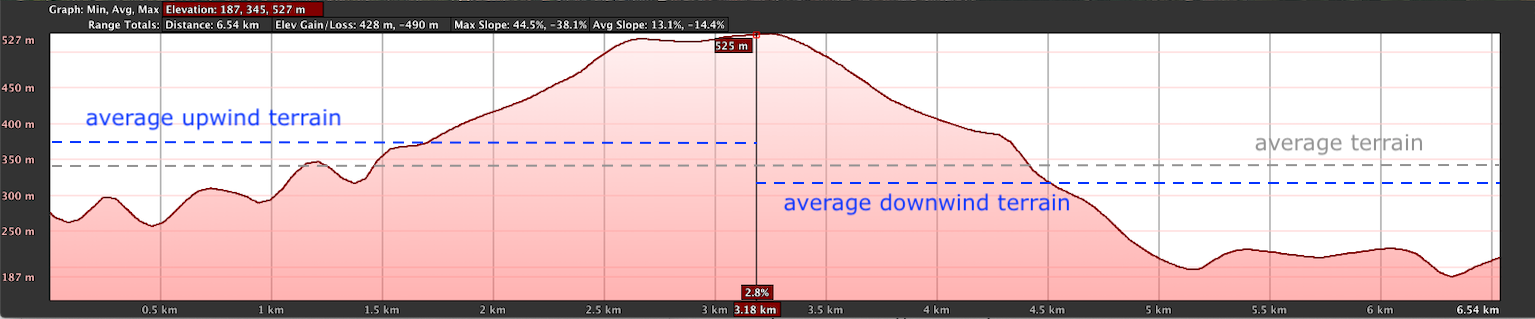
We will calculate $K_{zt}$ factor at the ground level at our site location, so $z=0$.  We can pick up our factors from the mentioned Tables 2-4 and 2-5. However, we have to make a decision on the Exposure category of the surrounding areas. In a later blog post I will also explain how to assess the exposure category, but for now we consider the area as Exposure C. TIA-222-G had a category for exp. C. which was basically the rural areas. In TIA-222-H everything which cannot be classified as Exposure B. or D. goes to Exposure C.

Now is the time to decide if we are having any speed-up effects or not. To check this we have to follow the 4 criteria's that TIA-222-H provides us. These 4 checkpoints are listed above.

Verifying speed-up effect

Without listing all 4 conditions, we can conclude that we have 4 "yes".

1. The feature is isolated and unobstructed, and
2. It portrudes by a factor of two or more above the average terrain, and the slope exceeds 0.1, and
3. It's height is greater than 4.57m, and
4. Our tower is located on the upper half of the hill.

The first point is a bit subjective and in not every cases is so obvious. But for now, by looking at the elevation profile we can say that, yes, our feature is isolated and unobstructed. Our other 3 criteria fortunately are more objective (in a certain level), so we can make some calculation. I'll show below an approximation for the terrain regarding its slope and the average upwind level. It is important that we are averaging the upwind terrain and not the whole area under investigation. You may realise it on the below profile that our downwind terrain average level would be at a lower altitude than the upwind one.

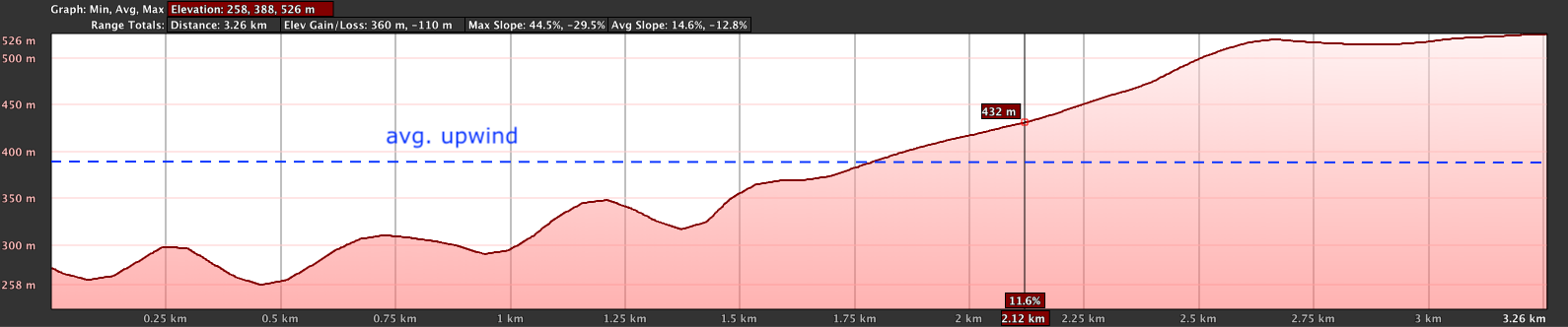
We can read the following measurements from the Google Earth Pro elevation profiles:

From here, we can calculate if the topography feature is portruding by a factor of two or not. In this case we are using the overall terrain, so the whole elevation profile average, and not only the upwind average.

$$ \Delta_{factor} = \frac{h_{max} - h_{min}}{h_{avg_(up)}-h_{min}}=\frac{526m - 258m}{345m-258m} = \frac{268}{87}= 3.08$$

Then, we need to also check the slope if exceeds 0.1. We can use the following equation, where $H$ is the height of the hill above the surrounding terrain, and $L$ is the length of the slope.

$$ \theta_{slope} = \frac{H}{L} = \frac{526m-388m}{2550m-1750m} = 0.17 $$
You may notice that I tried to use the location of the crest, so to get a realistic slope. You can also see on the screen shot that the average slope is around 14%, so we definitely have a speed up.

Now, we know that our topography feature is fulfilling all the requirements by the code, and by now we have all the values we need for the calculation of $K_{zt}$.

$ h = 15m $ this would be our tower height
$ K_c = 1.0 $ based on the Exposre category C. from Table 2-4.
$ K_t = 0.53 $ from Table 2-5, if our feature is a hill
$ H = 526m - 388m = 138m$, where 388m is the average upwind terrain
$ L = 800m $, the length of the upwind slope
$ f = 2.0 $ which is the height attenuation factor from Table 2-5.
First need to calculate $K_h$:
$$K_h=e^{ ( \frac{f \cdot z}{H} )}=2.718^{( \frac{2.0 \cdot 15}{138} )} = 1.24$$ (in case $h=0m$ so the ground level, then factor $K_h$ yields to 1.0)
Now,
$$ K_{zt} = [1+\frac{K_cK_t}{K_h} ]^2 = [1+\frac{1.0 \cdot 0.53}{1.24} ]^2 = 2.04$$

We are not calculating exact wind loads on any structure for now, but it worth noting where this factors goes in our wind pressure calculation. The basic formula to calculate wind velocity pressure is this:
$$ q_z = 0.613 \cdot K_z \cdot K_{zt} \cdot K_s \cdot K_e \cdot K_d \cdot V^2 $$
From here you may see that our velocity pressure would be multiplied by a factor of 2.04 due to the speed-up effect of our terrain. That's a significant increase in the wind forces which we have to apply to our structure. Let's proceed to Method 2, so to see what the rigorous procedure can result.

Notes: You may notice that we have skipped to check one "sub-condition" if speed-up occurs or not. I have taken it granted that we have to check the terrain features within a 2 miles radius (3.12km). But the code says that actually we have to do a check also within a radius equivalent of 100 times the feature height. This part is fairly tricky and I will show you at the end of the post why. The major challenge here is that, we don't know beforehand what is a feature? Along an elevation path there can be more features than one, for instance. Or it can also happen that if we check the 2 miles radius there won't be any feature portruding by a factor of two or more. But if we see the closer area of our site we might notice significant feature which is eventually protruding by a factor of two. This exact phenomen will be demonstrated at the automated way section.

Method 2: Rigorous Topographic Factor Procedure

Here we have more types of terrain features. This is due to the fine-tuned wind models from more researches and wind tunnel test. It found out for instance that the slope of the terrain has more impact in speed-up than the height for instance. The major difference compare to the TIA-222-G code is the flat-topped hill and flat-topped ridge as new added terrain feature types. You will see that how the flat-topped part will influence the $K_{zt}$ factor. This is similar to the equivalent embankment concept which was developed in the ESDU 91043 item.

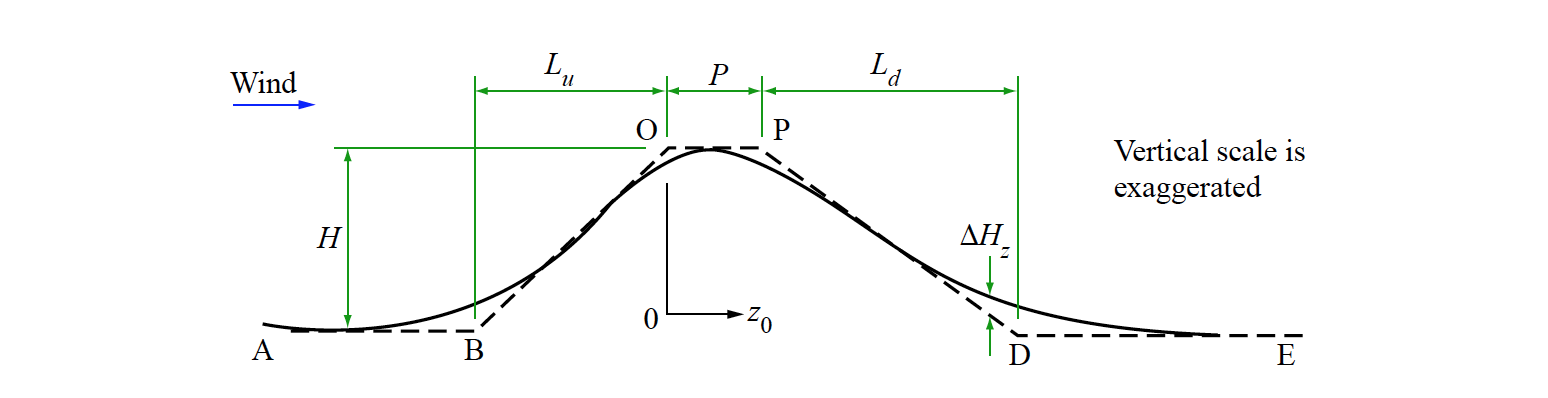
When we are moving away from "O", the upwind edge of our hill, towards right, the speed-up effect decreases. This phenomena has been implemented to the TIA-222-H code's Method 2, where we can take into account this effect through $K_2$ which you will see in a second. So let's see how it works in practise.

$K_{zt}$ shall be calcaulated by:
$$ K_{zt} = [ 1+K_1 \cdot K_2 \cdot K_3 ]^2$$
where,
$K_1=$ topographic feature factor adjusted for slope from Figure 2-1
$ = \beta K_{1'}$
$\beta=$ slope modifier from
$K_{1'}=$ topographic feature factor
$K_2=$ horizontal distance factor
$K_3=$ vertical distance factor

The topographic feature factor: $K_1$

The slope modifier $\beta$ can be computed as:$ \beta = 4 \cdot (\frac{H}{L})$, since $ \frac{1}{10} \leqslant \frac{H}{L} < \frac{1}{4}$
So from here,
$$ \beta = 4 \cdot (\frac{138}{800}) = 0.69 $$
then,
$$ K_1 = \beta \cdot K_{1'} = 0.69 \cdot 0.48 = 0.33 $$
Please note that our $K_{1'}$ value was fetched from Figure 2-1 in the standard, where I have chosen Flat Topped Hill as feature type. From the image above, the Approximation of the terrain shows more a flat-topped hill than a hill. Based on this, $K_{1'}$ was chosen as 0.48 as for exposure category C.

The horizontal distance factor: $K_2$

In the previous method we have had no chance to consider a horizontal distance, $x$ between the structure and crest. To be consistent, we will follow the same principal, so $x$ will be considered as $0$. It worth noting that if we consider the flat-topped hill and a certain distance for $x$, then we will get a reduction on the final $K_{zt}$. But this I leave it for you as an experiment ;)
$K_2$ can be calculated as below, based on the $\frac{H}{L}$ which in our case is 0.17:
$$ K_2 = 1-\left( \frac{4x}{3L} \right) = 1-\left( \frac{4 \cdot 0m}{3 \cdot 900m} \right) = 1.0 $$

The Vertical distance factor: $K_3$

$K_3$ can be calculated by the following formula, considering the same $\frac{H}{L}$ ratio:
$$ K_3 = e^{ \frac{-8z}{L} } = 2.718^{ \frac{-8 \cdot 15m}{800m} } = 0.86$$

Tip: It is always a good engineering practice that we perform all calculations with units. Then we get an immediate feedback if we did something wrong, or at least we can make sure that the formula we are using is working.

The topographic factor: $K_{zt}$

Now we can revisit our basic formula having all components to compute it.
$$ K_{zt} = [ 1+K_1 \cdot K_2 \cdot K_3 ]^2$$
then,
$$ K_{zt} = [ 1+ 0.33 \cdot 1.0 \cdot 0.86 ]^2 = 1.65 $$
Only for the curious, if we would choose a topographic feature type as "hill" instead of the "flat-topped hill" $K_{zt}$ would yield to 1.741. That is due to the different $K_{1'}$ factor.

Conclusion

We can notice that there is quite a significant difference between the two methods. There are different designations in the formulas between the two methods, but there are similar functions of them. There is one factor responsible for the shape or slope of the feature, and another one for the vertical distance. However, even the easier comparable vertical distance factor gives different result. What we can learn from here is that Method 1. more conservative than Method 2. Since Method 1. is identical of the procedure in TIA-222-G it is not a surprise. This how standards evolves and brings (most of the times) less conservative designs. In engineering, when we gain more and more knowledge, we can be more confident to offer more optimal solutions to the public without compromising safety.

The automated way

In case you want to be more efficient and avoid doing topography assessment all by hand, w have a solution. In TowerUp we have implemented the latest code of TIA-222-H and the underlying ASCE7-16 code to make this process automated. Only input you need is the site location coordinates for your area of interest. All the rest will be handled by our algorithm.

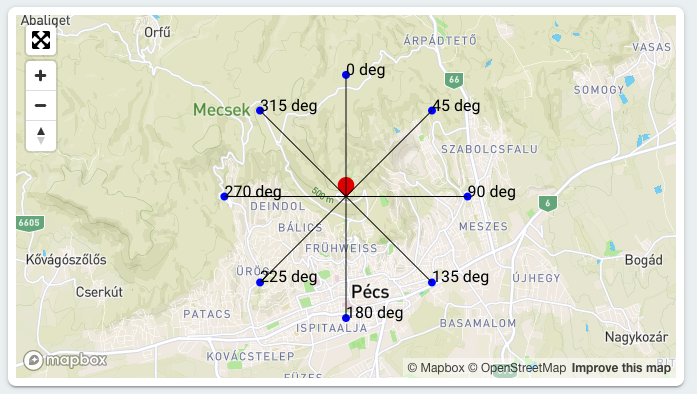
100 times the feature height

I have mentioned one shortcut I have made in the hand calculation about the conditions to fulfill in the code at section 2.6.6.1. To demonstrate the challange we take a look at our site but at this time our attention goes to direction 0 degree (N) and 315 deg (NW).

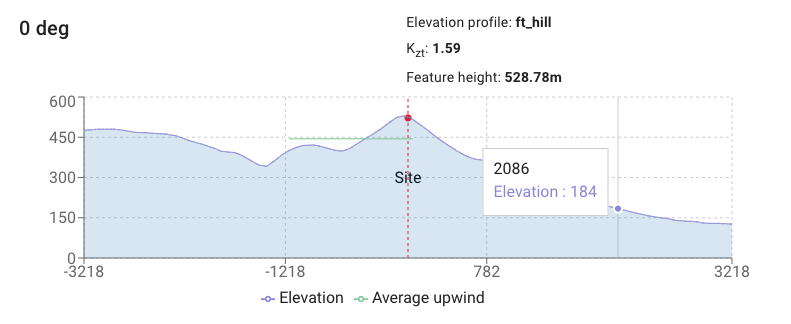
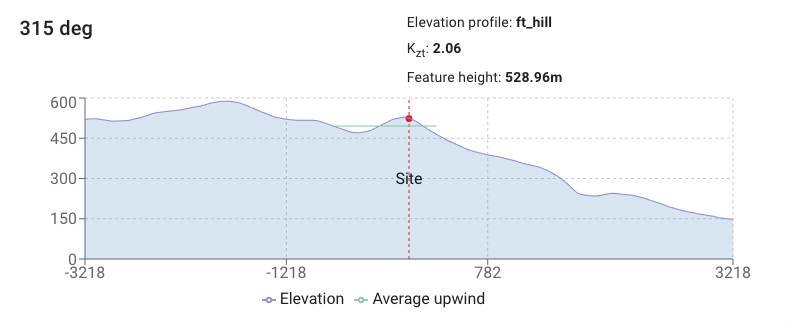
If we would use our engineering judgment (or at least if I use mine) I wouldn't assume significant feature within the upwind zone from direction 0 deg, and there is even a walley which would not be our concern to speed-up any wind velocity. Similarly to direction 315 deg. But the program detected a region within the radius of the 100 times of a feature height is governing the assessment. The solution is focusing on the most significant $K_{zt}$ factor. In order to be able to do this we have to perform hundreds of iterations through an elevation path. This would be very tedious to do it by hand, but at best, it wouldn't be practical at all. This was just one of challenges which leads us to develop an application which can help engineers to perform the task.